The Standard Normal Distribution

An important fact about normal random variables is that if X is normally distributed with parameters $\mu$ and $\sigma^2$, then $Y=\alpha X+\beta$ is normally distributed with parameters $\alpha\mu +\beta$ and $\alpha^2\sigma^2$. This follows because FY, the cumulative distribution function of the random variable Y, is given, when $\alpha >0$, by

$\begin{array}{rcl} F_Y(a)&=&P\{Y\leq a\} \\ \\ &=&P\{\alpha X +\beta\leq a\} \... ...-\frac{[y-(\alpha\mu+\beta)]^2}{2\alpha^2\sigma^2}\right\}dy \\ \\ \end{array}$

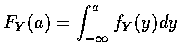Since, $F_Y(a)=\displaystyle\int_{-\infty}^{a}f_Y(y)dy$, that the probability density function of Y, FY(y), is given by

$\displaystyle f_Y(y)=\frac{1}{\displaystyle\sqrt{2\pi}\alpha\sigma} \exp\left\{-\frac{[y-(\alpha\mu+\beta)]^2}{2\alpha^2\sigma^2}\right\}dy$
Hence Y is normally distributed with parameters $\alpha\mu +\beta$ and $(\alpha\sigma)^2$

If X is normally distributed with parameters $\mu$ and $\sigma^2$, then $Z=(X-\mu)/\sigma$ is normally distributed with parameters 0 and 1. Such a random variable Z is said to have the standard, or unit, normal distribution. It is traditional to denote the cumulative distribution function of a standard normal random variable by

$\Phi (x)=\displaystyle\frac{1}{\displaystyle\sqrt{2\pi}}\int_{-\infty}^{x}e^{-y^2/2}dy$
and we have $\Phi (-x)=1-\Phi (x)\qquad -\infty<x<\infty$.

If Z is a standard normal random variable, then

$P\{Z\leq -x\}=P\{Z>x\}\qquad -\infty<x<\infty$
Since $Z=(X-\mu)/\sigma$ is standard normal random variable whenever X is normally distributed with parameters $\mu$ and $\sigma^2$, it follows that the distribution function of X can be expressed as
$\begin{array}{rcl} F_x(a)&=&P\{X\leq a\} \\ \\ &=&P\left (\displaystyle\frac{X... ... \\ &=&\Phi\left (\displaystyle\frac{a-\mu}{\sigma}\right ) \\ \\ \end{array}$