Multinomial Coefficients 多項式係數

Notation
If $n_1+n_2+\cdots+n_r=n$, we define $\displaystyle{n\choose {n_1,n_2,\cdots ,n_r}}$ by

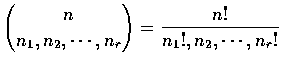
$\displaystyle{n\choose {n_1,n_2,\cdots ,n_r}}=\frac{n!}{n_1!,n_2,\cdots,n_r!}$
Thus $\displaystyle{n\choose {n_1,n_2,\cdots ,n_r}}$ represents the number of possible divisions of n distinct objects into r distinct group of respective sizes $n_1,n_2,\cdots ,n_r$. The numbers are known as multinomial coefficients.

The Multinomial Theorem

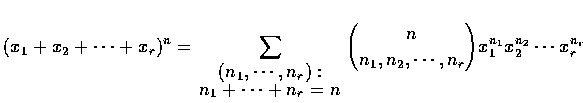
$\displaystyle(x_1+x_2+\cdots +x_r)^n= \sum\limits_{\displaystyle(n_1,\cdots ,n_... ...ts +n_r=n} {n\choose {n_1,n_2,\cdots ,n_r}} x_1^{n_1} x_2^{n_2}\cdots x_r^{n_r}$

That is, the sum is over all nonnegative integer-valued vectors $(n_1,n_2,\cdots ,n_r)$ such that $n_1+n_2+\cdots+n_r=n$.

Example
$\displaystyle\begin{array}{rcl} (x_1+x_2+x_3)^2&=&{2\choose {2,0,0}}x_1^2x_2^0x... ...2^2+x_3^2+2x_1x_2+2x_1x_3+2x_2x_3 \end{array} \qquad\rule[0.02em]{1.0mm}{1.5mm}$