δΎ‹
Consider a population with mean = 82 and standard deviation = 12.
(a)
If a random sample of size 64 is selected, what is the probability that the sample mean will lie between 80.8 and 83.2?
(b)
With a random sample of size 100, what is the probability that the sample mean will lie between 80.8 and 83.2?

(a)
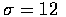We have $\mu=82$ and $\sigma=12$. Since n=64 is large, the central limit theorem tells us that the distribution of $\overline{X}$ is approximately normal with

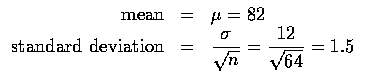
$\begin{array}{rcl} \mbox{mean}&=&\mu=82 \\ \mbox{standard deviation}&=&\displaystyle\frac{\sigma}{\sqrt{n}}= \frac{12}{\sqrt{64}}=1.5 \\ \end{array}$

To calculate $P[80.8<\overline{X}<83.2]$, we convert to the standardized variable

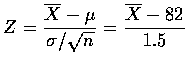
$\displaystyle Z=\frac{\overline{X}-\mu}{\sigma/\sqrt{n}}=\frac{\overline{X}-82}{1.5}$

The z-values corresponding to 80.8 and 83.2 are

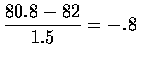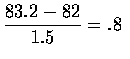
$\displaystyle\frac{80.8-82}{1.5}=-.8$ and $\displaystyle\frac{83.2-82}{1.5}=.8$

Consequently,

$\begin{array}{rcl} P[80.8<\overline{X}<83.2]&=&P[-.8<Z<.8] \\ &=& .7881-.2119 \mbox{ (using the normal table) } \\ &=&.5762 \\ \end{array}$

(b)
We now have n=100, so 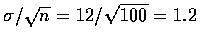$\sigma/\sqrt{n}=12/\sqrt{100}=1.2$,and
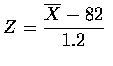
$\displaystyle Z=\frac{\overline{X}-82}{1.2}$

Therefore,

$\begin{array}{rcl} P[80.8<\overline{X}<83.2]&=&\displaystyle P[\frac{80.8-82}{1... ...-82}{1.2}] \\ &=&P[-1.0<Z<1.0] \\ &=&.8413-.1587 \\ &=&.6826 \\ \end{array}$

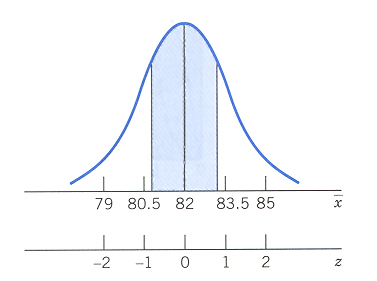
Note that the interval (80.8, 83.2) is centered at $\mu=82$. The probability that $\overline{X}$ will lie in this interval is larger for n=100 than for n=64.