Simpson's Paradox

Quite surprising and misleading conclusions can occur when data from different sources are combined into a single table. We illustrate this reversal of implications with graduate school admissions data.

例 2
We consider graduate school admissions at a large midwestern university but, to simplify, we use only two departments as the whole school. We are interested in comparing admission rates by gender and obtain the following data for the school.

Table 4 School Admission Rates

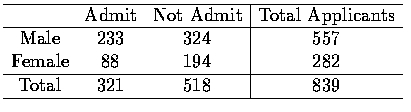
$\begin{array}{ccc\vert c} \hline &\mbox{Admit}&\mbox{Not Admit}&\mbox{Total App... ...box{Female}&88&194&282 \\ \hline \mbox{Total}&321&518&839 \\ \hline \end{array}$

It is clear from these admission statistics that the proportion of males admitted, 233/557=.418, is greater than the proportion of females admitted, 88/282=.312.

Does this imply some type of discrimination? Not necessarily. By checking the admission records, we were able to further categorize the cases according to department in Table5. Table 4 is the aggregateof these two sets of data.

Table 5 Admission Rates by Department

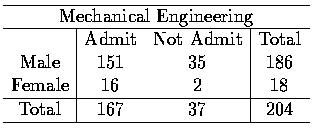
$\begin{array}{c\vert cc\vert c} \hline \multicolumn{4}{c}{\mbox{Mechanical Engi... ... \mbox{Female}&16&2&18 \\ \hline \mbox{Total}&167&37&204 \\ \hline \end{array}$

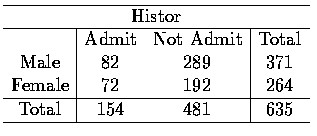
$\begin{array}{c\vert cc\vert c} \hline \multicolumn{4}{c}{\mbox{Histor}} \\ \hl... ...box{Female}&72&192&264 \\ \hline \mbox{Total}&154&481&635 \\ \hline \end{array}$

One of the two departments, mechanical engineering, has mostly male applicants. Even so the proportion of males admitte, 151/186=.812, is smaller than the proportion of females admitted, 16/18=.889. The same is true for the history department where the proportion of males admitted, 82/371=.221, is again smaller than the proportion of females admitted, 72/264=.273. When the data are studied department by department, the reverse conclusion holds; females have a higher admission rate in both cases!

To obtain the correct interpretation, these data need to be presented as the full three-way table of gender-admission action-department as given above. If department is ignored and the data aggregated acros this variable, "department" can act as an unrecorded or lurking variable. In this example, it has reversed the direction of possible gender bias and led to the erroneous conclusion that males have a higher admission rate than females.

The reversal of the comparison, such as in 例 2, when dataarecombined from several groups is called Simpson's paradox.

When data from several sources are aggregated into a single table, there is always the danger that unreported variables may cause a reversal of the findings. In practical applications, there is not always agreement on how much effort to expend following up on unreported variable. When comparing two medical treatments, the results often need to be adjusted for the age, gender, and sometimes current health of the subjects and other variables.